Teoria de Morse para presentaciones de grupos &
aplicaciones a persistencia
XIMENA FERNANDEZ
University of Oxford
UK CENTRE FOR TOPOLOGICAL DATA ANALYSIS
Coloquio Iberoamericano de Álgebra y Teoría de Nudos
'Every mathematician has a secret weapon.
Mine is Morse theory.'
Raoul Bott.
Motivation
CW-complexes of dim 2
Group presentations
CW-complexes of dim 2
$K_{\mathcal{P}}$
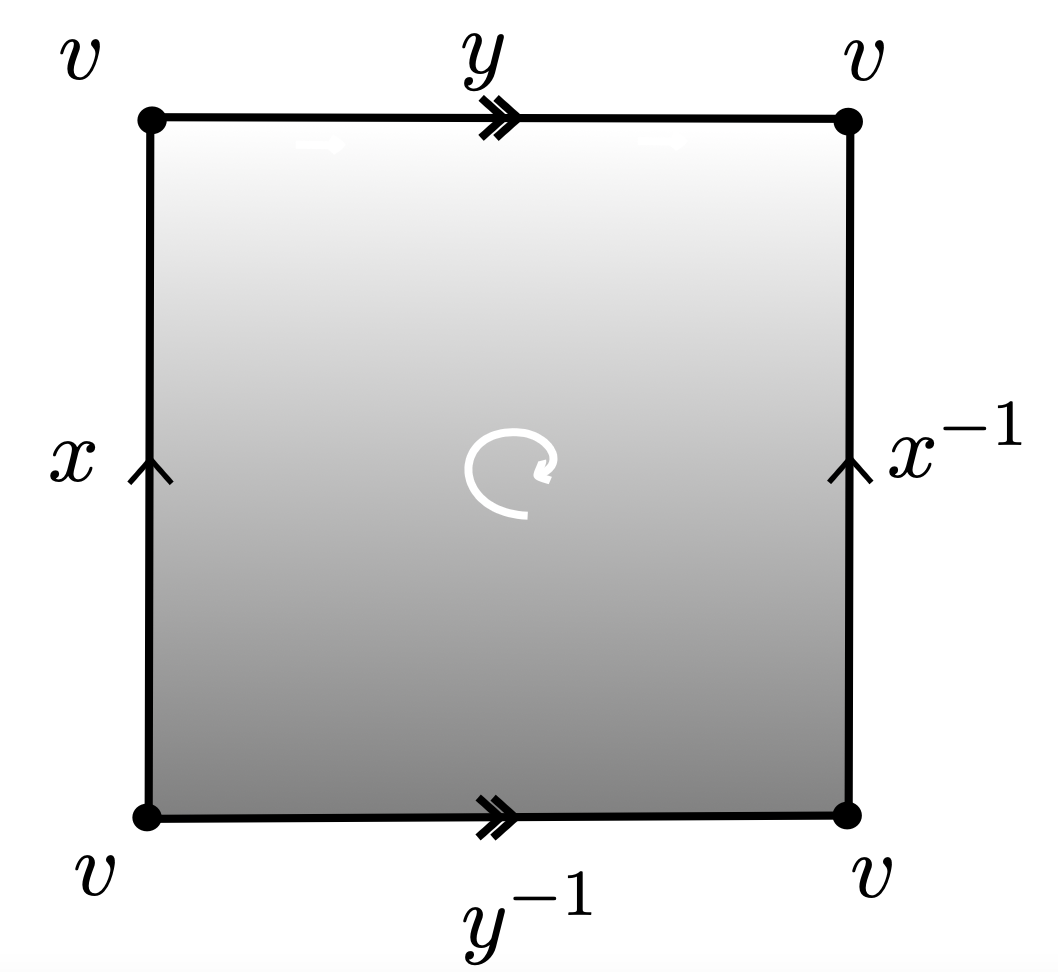
Group presentations
$\mathcal{P}= \langle x,y ~|~xyx^{-1}y^{-1}\rangle $
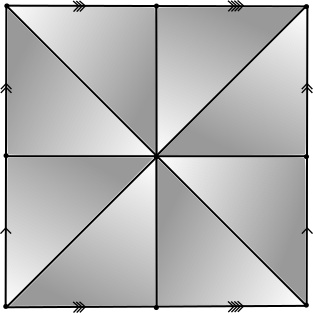
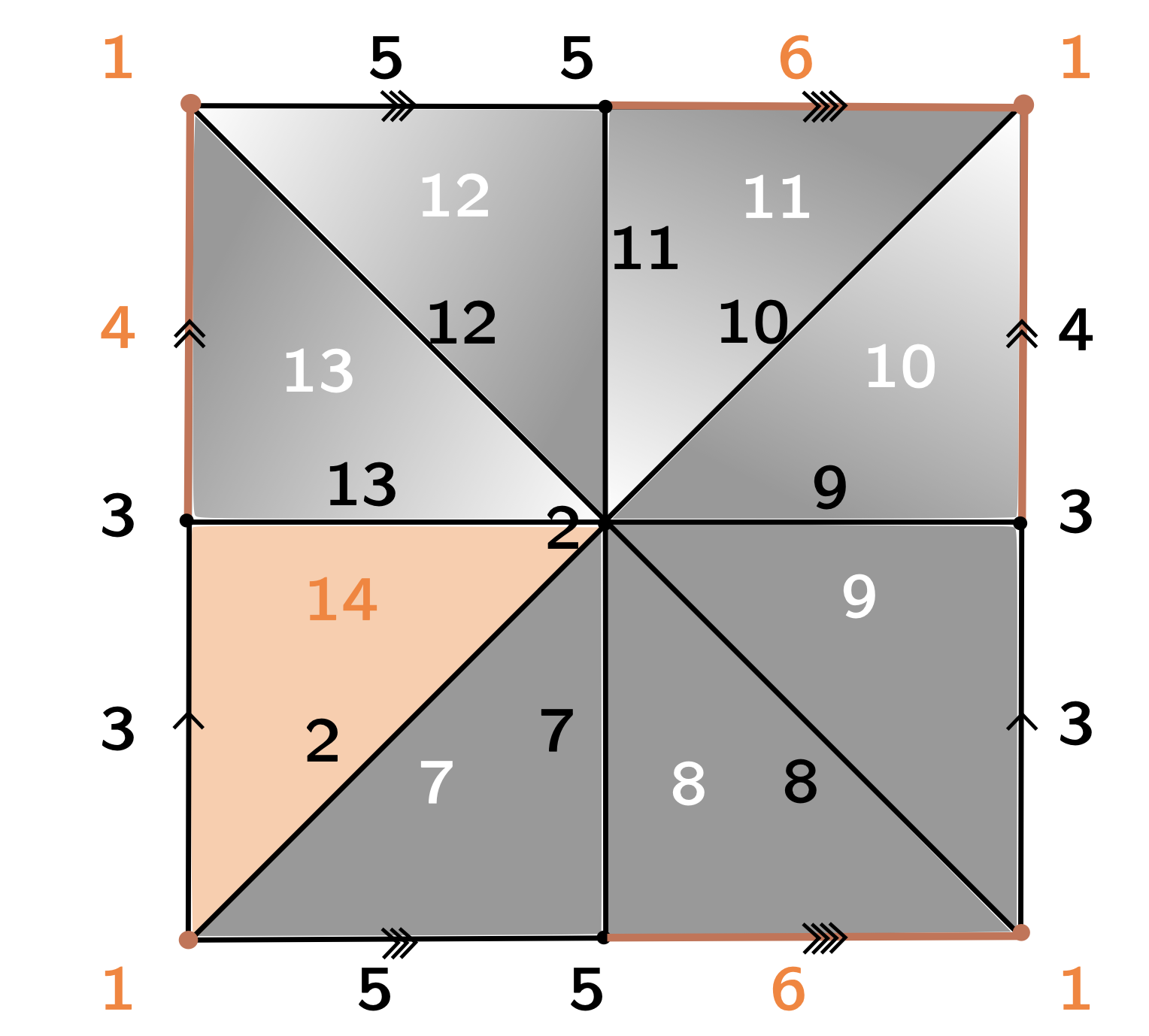
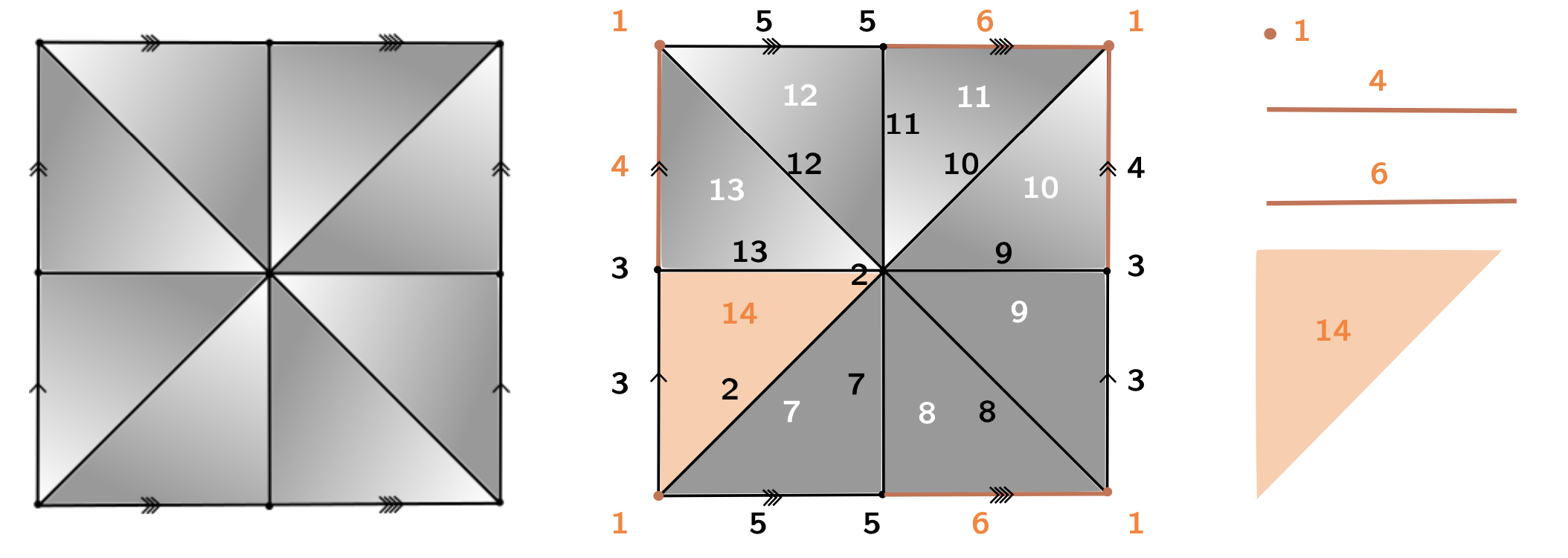
CW-complexes of dim 2
$K$

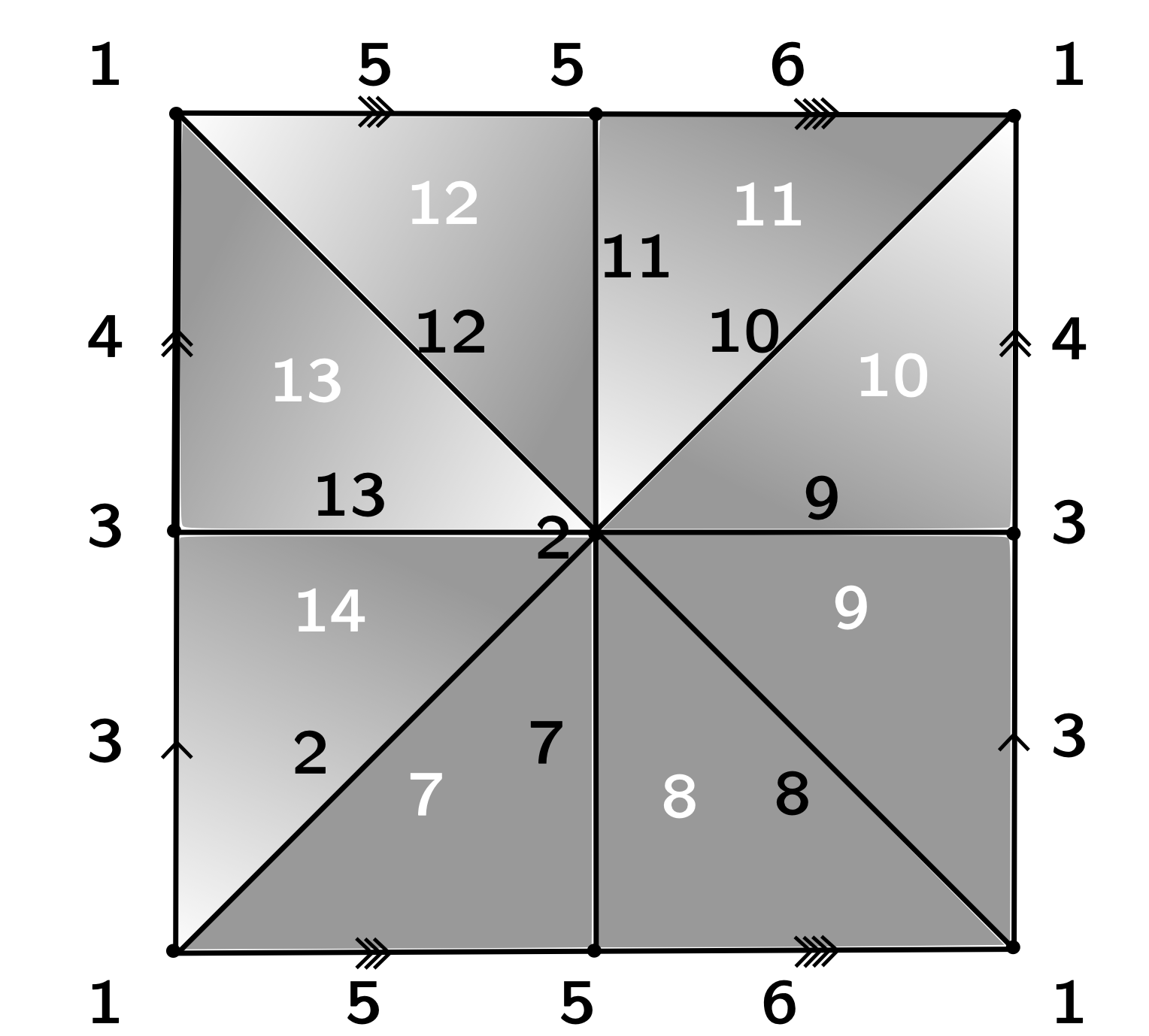
Group presentations
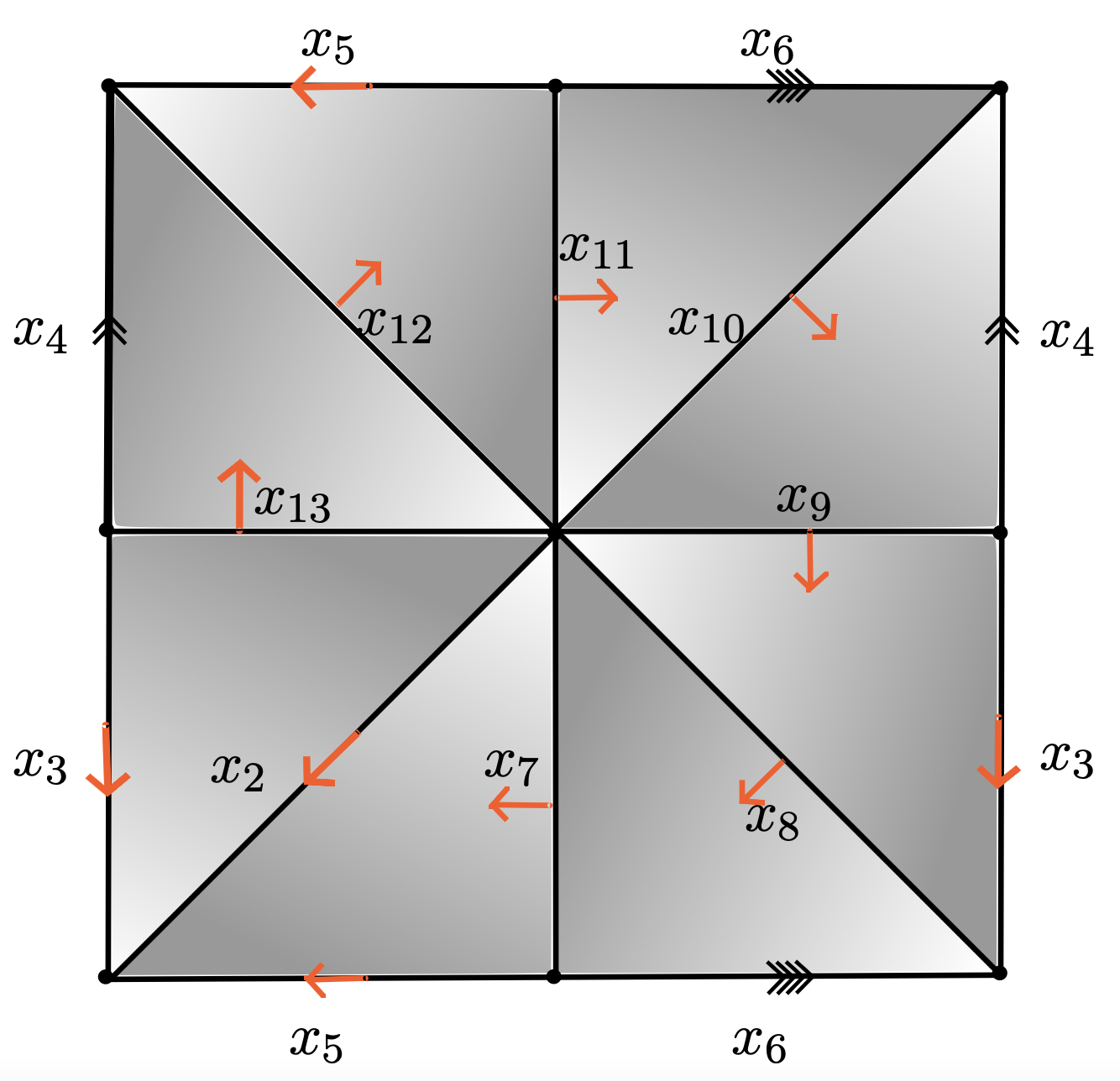
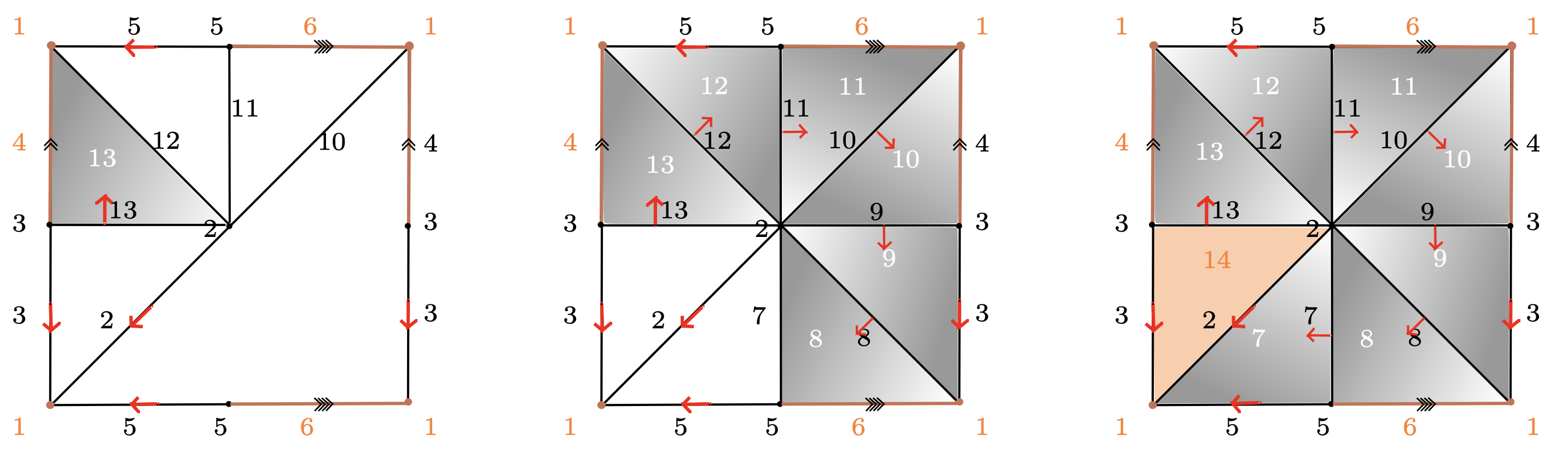
$\mathcal{P}_K = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | \\~ ~ ~ ~ ~ ~ ~ ~ ~ ~x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, \\ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
Combinatorial Homotopy Theory
Combinatorial Homotopy Theory
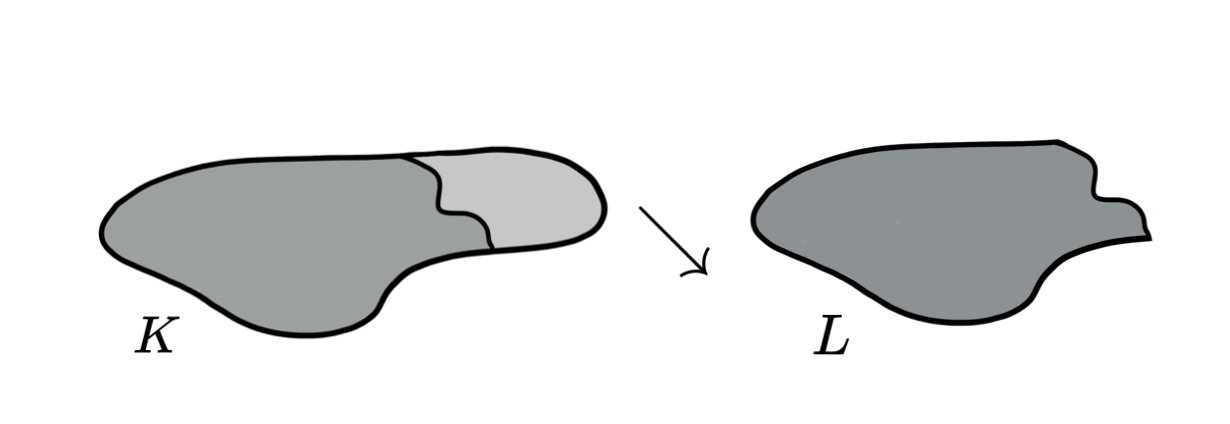
Let $K,L$ be CW-complexes.
- There is an elementary collapse $K\searrow^{\hspace{-5pt}e} L$ (or elementary expansion $L^{\hspace{5pt}e}\hspace{-8pt}\nearrow K$)
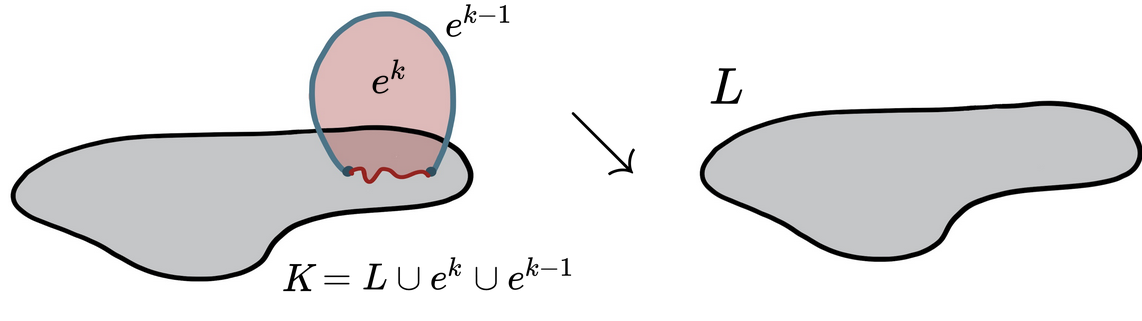
Combinatorial Homotopy Theory
Let $K,L$ be CW-complexes.
- A collapse $K\searrow L$ is an ordered pairing $\{(e_i, x_i):1\leq i \leq k\}$ of the cells in $K\smallsetminus L$ such that if $K_i = L \cup \bigcup_{j < i}e_j \cup \bigcup_{j < i}x_j$, then $K_i\searrow^{\hspace{-5pt}e} K_{i-1}$.
Simple Homotopy Theory
Let $K,L$ be CW-complexes.
- There is an $n$-deformation $K \nearrow \hspace{-2 pt}\searrow^{^{\hspace{-5pt}n}}L$ if there is a sequence of CW-complexes $K=K_0,K_1,\dots K_r=L$ such that $K_i \searrow^{\hspace{-5pt}e} K_{i+1}$ or $K_i ^{\hspace{5pt}e}\hspace{-5pt}\nearrow K_{i+1}$ for each $0\leq i\leq r-1$, and $\dim(K_i)\leq n$ for all $1\leq i\leq r$.
- There is simple homotopy equivalence $K\nearrow\hspace{-2 pt}\searrow L$ if $K \nearrow \hspace{-2 pt}\searrow^{^{\hspace{-5pt}n}}L$ for some $n\in \mathbb{N}$.
Simple Homotopy Theory
Let $K,L$ be CW-complexes.
- $K \nearrow\hspace{-2 pt}\searrow L \Rightarrow K\simeq L.$
- The converse is not true, the obstruction is measured by the Whitehead group.
If the Whitehead group $\mathrm{Wh} (K)$ of the complex $K$ is trivial, then any complex homotopy equivalent to $K$ is also simple homotopy equivalent to $K$. - For $K$ contractible, $\mathrm{Wh}(K)=0$ and hence $K\simeq * \Leftrightarrow K \nearrow\hspace{-2 pt}\searrow *.$
- (Whitehead '50) If $K, L$ are $n$-complexes, then $K \nearrow\hspace{-2 pt}\searrow L \Rightarrow K \nearrow\hspace{-2 pt}\searrow^{^{\hspace{-7pt}n+1}}L$ if $n\neq 2$.
For $n=2$, this question is open. - (C.T.C. Wall '66) If $K, L$ are $2$-complexes, then $K \nearrow\hspace{-2 pt}\searrow L \Rightarrow K\nearrow\hspace{-2 pt}\searrow^{^{\hspace{-7pt}4}} L$.
Discrete Morse Theory
Discrete Morse Theory
Goal: 'Simplify' the cell decomposition of a CW-complex while preserving its homotopy type.
- $K$ a regular CW-complex.
Discrete Morse Theory
Goal: 'Simplify' the cell decomposition of a CW-complex while preserving its homotopy type.
- $K$ a regular CW-complex.
- $f:K\to \mathbb{R}$ a discrete Morse function.
For every cell $e^n$ in $K$, $\#\{e^n\succ e^{n-1}: f(e^n)\leq f(e^{n-1})\}\leq 1 \text{ and } \#\{e^n\prec e^{n+1} : f(e^n)\geq f(e^{n+1})\}\leq 1.$
Discrete Morse Theory
Goal: 'Simplify' the cell decomposition of a CW-complex while preserving its homotopy type.
- $K$ a regular CW-complex.
- $f:K\to \mathbb{R}$ a discrete Morse function.
- $C$ the set of critical cells of $K$.
An $n$-cell $e^n \in K$ is a critical cell of index $n$ if the values of $f$ in every face and coface of $e^n$ increase with dimension.
Discrete Morse Theory
Goal: 'Simplify' the cell decomposition of a CW-complex while preserving its homotopy type.

Discrete Morse Theory
Goal: 'Simplify' the cell decomposition of a CW-complex while preserving its homotopy type.
- $K$ a regular CW-complex.
- $f:K\to \mathbb{R}$ a discrete Morse function.
- $C$ the set of critical cells of $K$.
Theorem [Forman, '98]. $K$ is homotopy equivalent to a CW-complex $K_{\mathcal{M}}$ with exactly one cell of dimension $k$ for every critical cell of index $k$.
Discrete Morse Theory
&
Simple Homotopy Theory
Discrete Morse Theory & Simple Homotopy Theory
Lemma (Internal collapse): Let $K$ be a CW-complex of dimension $\leq n$. Let $\varphi:\partial D^n\to K$ be the attaching map of an $n$-cell $e^n$. If $K \searrow L$, then \[K\cup e^n \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}}L\cup \widetilde{e}^n\] where the attaching map $\widetilde{\varphi}\colon \partial D^ n\to L$ of $\widetilde{e}^n$ is defined as $\widetilde{\varphi}=r \varphi$ with $r:K\to L$ the canonical strong deformation retract induced by the collapse $K \searrow L$.
Discrete Morse Theory & Simple Homotopy Theory
Lemma (Internal collapse): Let $K$ be a CW-complex of dimension $\leq n$. Let $\varphi:\partial D^n\to K$ be the attaching map of an $n$-cell $e^n$. If $K \searrow L$, then \[K\cup e^n \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}}L\cup \widetilde{e}^n\] where the attaching map $\widetilde{\varphi}\colon \partial D^ n\to L$ of $\widetilde{e}^n$ is defined as $\widetilde{\varphi}=r \varphi$ with $r:K\to L$ the canonical strong deformation retract induced by the collapse $K \searrow L$.
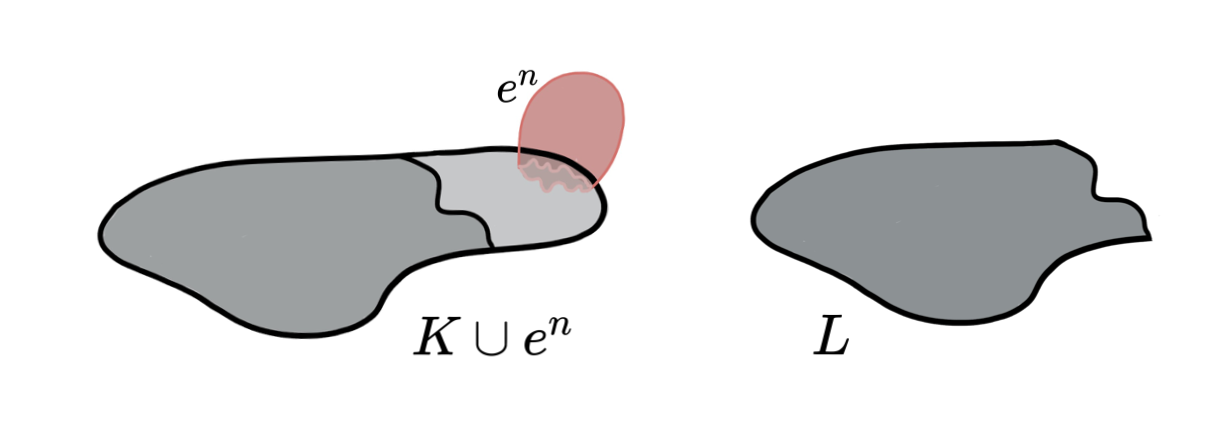
Discrete Morse Theory & Simple Homotopy Theory
Lemma (Internal collapse): Let $K$ be a CW-complex of dimension $\leq n$. Let $\varphi:\partial D^n\to K$ be the attaching map of an $n$-cell $e^n$. If $K \searrow L$, then \[K\cup e^n \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}}L\cup \widetilde{e}^n\] where the attaching map $\widetilde{\varphi}\colon \partial D^ n\to L$ of $\widetilde{e}^n$ is defined as $\widetilde{\varphi}=r \varphi$ with $r:K\to L$ the canonical strong deformation retract induced by the collapse $K \searrow L$.
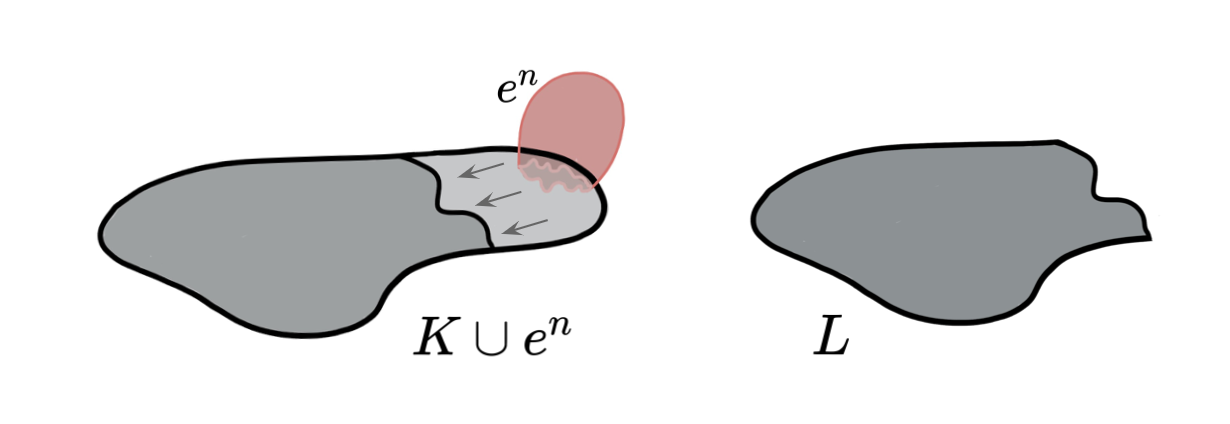
Discrete Morse Theory & Simple Homotopy Theory
Lemma (Internal collapse): Let $K$ be a CW-complex of dimension $\leq n$. Let $\varphi:\partial D^n\to K$ be the attaching map of an $n$-cell $e^n$. If $K \searrow L$, then \[K\cup e^n \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}}L\cup \widetilde{e}^n\] where the attaching map $\widetilde{\varphi}\colon \partial D^ n\to L$ of $\widetilde{e}^n$ is defined as $\widetilde{\varphi}=r \varphi$ with $r:K\to L$ the canonical strong deformation retract induced by the collapse $K \searrow L$.
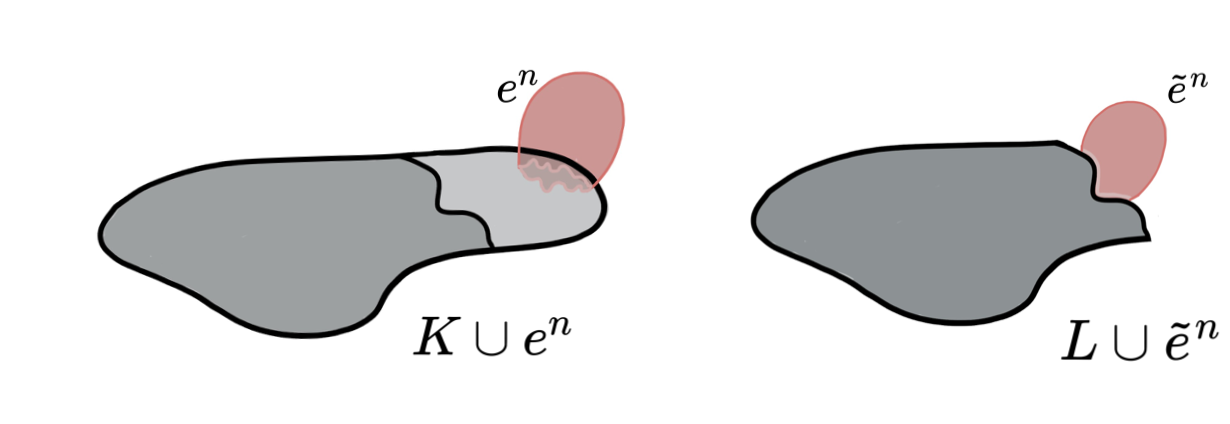
Discrete Morse Theory & Simple Homotopy Theory
Lemma (Internal collapse): Let $K$ be a CW-complex of dimension $\leq n$. Let $\varphi:\partial D^n\to K$ be the attaching map of an $n$-cell $e^n$. If $K \searrow L$, then \[K\cup e^n \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}}L\cup \widetilde{e}^n\] where the attaching map $\widetilde{\varphi}\colon \partial D^ n\to L$ of $\widetilde{e}^n$ is defined as $\widetilde{\varphi}=r \varphi$ with $r:K\to L$ the canonical strong deformation retract induced by the collapse $K \searrow L$.
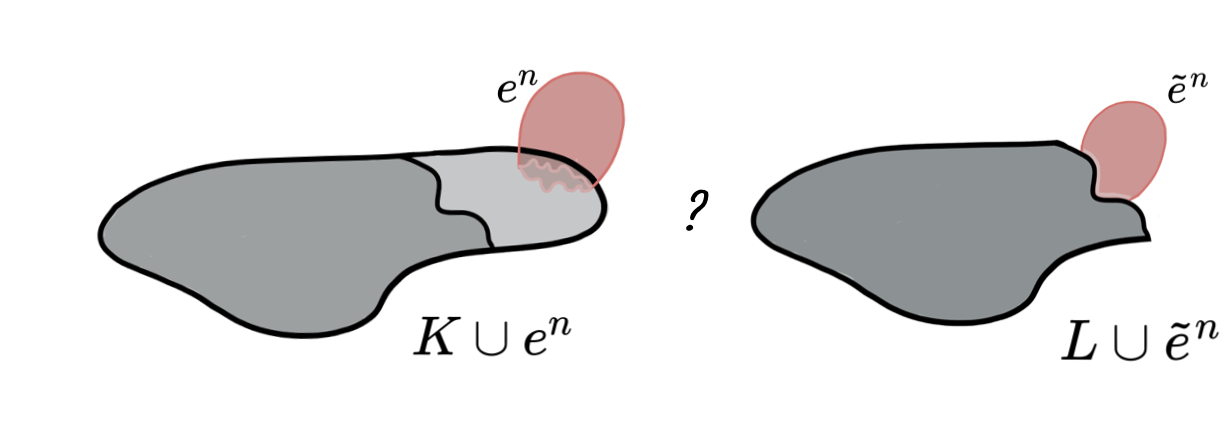
Discrete Morse Theory & Simple Homotopy Theory
Lemma (Internal collapse): Let $K$ be a CW-complex of dimension $\leq n$. Let $\varphi:\partial D^n\to K$ be the attaching map of an $n$-cell $e^n$. If $K \searrow L$, then \[K\cup e^n \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}}L\cup \widetilde{e}^n\] where the attaching map $\widetilde{\varphi}\colon \partial D^ n\to L$ of $\widetilde{e}^n$ is defined as $\widetilde{\varphi}=r \varphi$ with $r:K\to L$ the canonical strong deformation retract induced by the collapse $K \searrow L$.
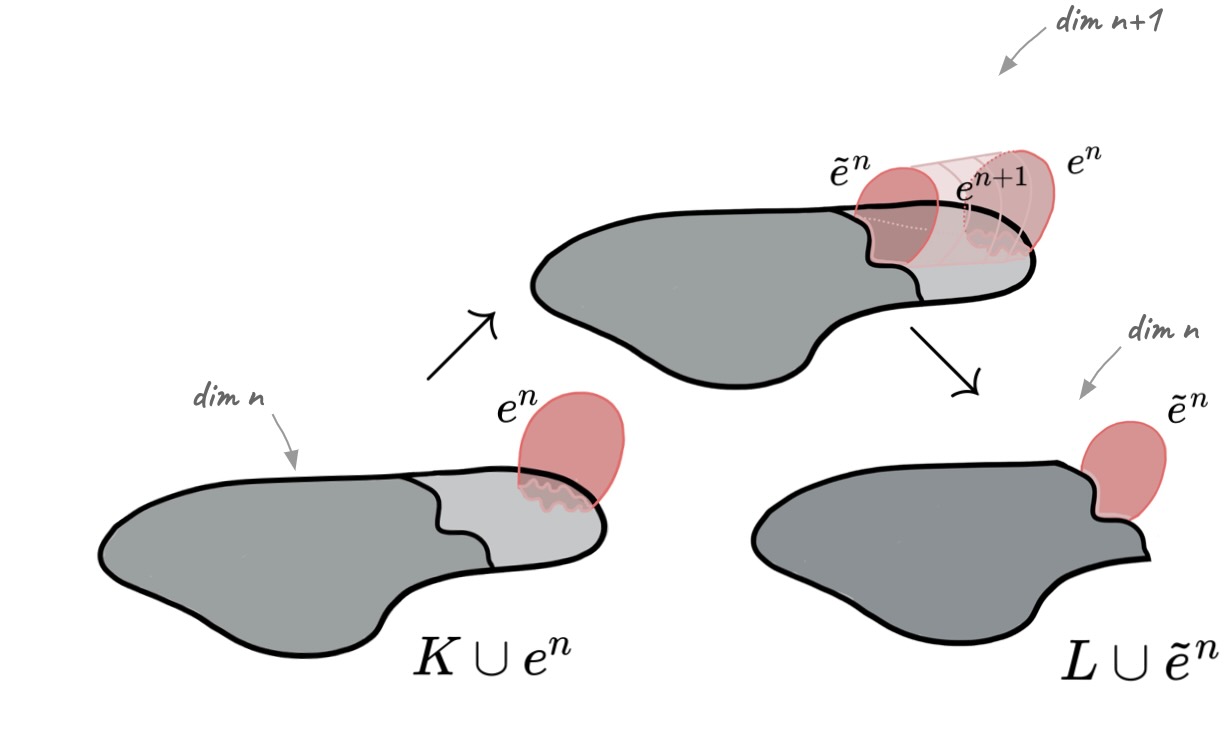
Discrete Morse Theory & Simple Homotopy Theory
Proposition [F.]: Let $ \displaystyle K \cup \bigcup_{i=1}^d e_i$ be a CW-complex where
$\dim (K) \leq \dim (e_{i})\leq \dim (e_{i+1})\leq n$ for all $i=1, 2, \dots, d$.
Let $\displaystyle \varphi_j:\partial D_j\to K\cup \bigcup_{i < j} e_i$ be the attaching map of $e_j$.
If $K\searrow L$, then there exist CW-complexes $Z_1\leq Z_2\leq \dots \leq Z_d$ of dim $\leq n+1$ such that for every $j=1, 2, \dots, d,$
\[K\cup \bigcup_{i=1}^j e_i \nearrow Z_j \searrow L\cup
\bigcup_{i=1}^j \widetilde e_i\] where the attaching map
$\displaystyle \widetilde \varphi_j:\partial D_j\to L\cup \bigcup_{i< j}
\widetilde e_i$ of the cell $\widetilde e_j$ is defined inductively as:
$\bullet ~~\displaystyle \widetilde \varphi_1=r_0\varphi_1$ with $r_0:K\to L$ the canonical strong deformation retract and for $j>1$,
$\bullet ~~\widetilde \varphi_{j}=\widetilde r_{j-1}\imath_{j-1}\varphi_{j}$ where $ \widetilde r_{j-1}:Z_{j-1}\to L\cup \bigcup_{i< j} \widetilde e_i$ is the strong deformation retract and $\imath_{j-1}: K\cup \bigcup_{i< j} e_i \to Z_{j-1}$ is the inclusion.
Discrete Morse Theory & Simple Homotopy Theory
Theorem [F.]: Let $K$ be a regular CW-complex of dim $n$ and let $f:K\to \mathbb{R}$ be discrete Morse function. Then, $f$ induces a sequence of internal collapses given by a filtration of $K$ \[ \varnothing = K_{-1} \subseteq L_0 \subseteq K_0\subseteq L_1\subseteq K_1 \dots \subseteq L_{N}\subseteq K_{N}=K\] such that $K_j\searrow L_{j}$ for all $1\leq j\leq N$ and $\displaystyle L_{j}=K_{j-1}\cup \bigcup_{i=1}^{d_j} e_i^j$ with $\{e_i^j:0\leq j\leq N, 1\leq i \leq d_j\}$ the set of critical cells of $f$. Moreover, \[ K \nearrow\hspace{-1.5 pt} \searrow^{^{\hspace{-8pt} n+1}} L_0\cup \bigcup_{j=1}^{N} \bigcup_{i=1}^{d_j}\widetilde e_i^j = K_\mathcal{M}.\]
* Here, the attaching maps of the cells $\widetilde e_i^j$ can be explicitly reconstructed from the internal collapses.
Discrete Morse Theory & Simple Homotopy Theory

Morse theory for
group presentations
CW-complexes of dim 2
Group presentations
CW-complexes of dim 2
$K$

Group presentations
$\mathcal{P}_K = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | \\~ ~ ~ ~ ~ ~ ~ ~ ~ ~x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, \\ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
Morse theory for group presentations
$K$

$\mathcal{P}_K = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | \\~ ~ ~ ~ ~ ~ ~ ~ ~ ~x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, \\ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$K + f\colon K\to \mathbb{R}$ Morse function
$\mathcal{P}_{K_{\mathcal{M}}} = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $
Morse theory for group presentations
Given $K$ a regular CW-complex of dim 2 and $f\colon K\to \mathbb{R}$ a discrete Morse function with a single critical 0-cell, we developed an algorithm to compute the Morse presentation $\mathcal{P}_{K_{\mathcal M}}$.
$O(M^2)$ with $M$ = |2-cells of $K$|
ximenafernandez/Finite-Topological-Spaces (SAGE)
Posets-Package (GAP) (joint with K. Piterman & I. Sadofschi Costa).
(c.f. Brendel, Ellis, Juda, Mrozek. Fundamental group algorithm for low dimensional tessellated CW-complexes, 2015. arXiv:1507.03396)
Morse theory for group presentations
Given $K$ a regular CW-complex of dim 2 and $f\colon K\to \mathbb{R}$ a discrete Morse function with a single critical 0-cell, we developed an algorithm to compute the Morse presentation $\mathcal{P}_{K_{\mathcal M}}$.
The Andrews-Curtis conjecture
The Andrews-Curtis conjecture
Conjecture [Andrews & Curtis, 1965]. Any finite balanced presentation $\mathcal{P}=\langle x_1,\dots,x_n ~|~ r_1,\dots,r_n\rangle $ of the trivial group can be transformed into the empty presentation $\langle ~|~\rangle$ by a finite sequence of the following operations:
- replace some relator $r_i$ by $r_i^{-1}$
- replace some relator $r_i$ by $r_i r_j$ for some $j \neq i$
- replace some relator $r_i$ by a conjugate $wr_i w^{-1}$ for some $w$ in the free group $F(x_1, x_2, \dots, x_n)$
- add a generator $x_{n+1}$ and a relator that coincides with $x_{n+1}$, or the inverse of this operation
Tietze transformations
Theorem [Tietze, 1908]. Any finite presentation $\mathcal{P}=\langle x_1,\dots,x_n ~|~ r_1,\dots,r_m\rangle $ of a group $G$ can be transformed into any other presentation of the same group by a finite sequence of the following operations:
- replace some relator $r_i$ by $r_i^{-1}$
- replace some relator $r_i$ by $r_i r_j$ for some $j \neq i$
- replace some relator $r_i$ by a conjugate $wr_i w^{-1}$ for some $w$ in the free group $F(x_1, x_2, \dots, x_n)$
- add a generator $x_{n+1}$ and a relator that coincides with $x_{n+1}$, or the inverse of this operation
- add a relator $1$, or the inverse of this operation
The Andrews-Curtis conjecture
Potential counterexamples.
- $\mathcal{P}=\langle x, y~|~ xyx = yxy,~ x^n = y^{n+1}\rangle,~n\geq 2~$ [Akbulut & Kirby, 1985]
- $\mathcal{P} = \langle x,y~|~x^{-1}y^n x = y^{n+1}, ~x = y^{-1}xyx^{-1}\rangle,~n\geq 2~ $ [Miller & Schupp, 1999]
- $\mathcal{P}=\langle x,y~| ~x=[x^m,y^n], y=[y^p,x^q]\rangle ,~n,m,p,q\in \mathbb{Z}~$ [Gordon, 1984]
Theorem [Bridson, 2015] There exist balanced presentations of the trivial group that satisfy the AC-conjecture for which the minimun length of any simplification sequence is superexponential in the total length of the relators.
The Andrews-Curtis conjecture
Remark [Referee]. AC-transformations of group presentations are in correspondence with 3-deformations of 2-complexes.

Morse theory for group presentations
Corollary [F. 2017]. Let $\mathcal{P}$ be a finite presentation of a finitely presented group. Let $K'_{\mathcal{P}}$ be the barycentric subdivision of the standard complex $K_{\mathcal{P}}$ and let $f:K'_{\mathcal{P}}\to \mathbb{R}$ be a discrete Morse function.
Then $\mathcal{P}\sim_{AC}\mathcal{P}_{(K'_{\mathcal{P}})_{\mathcal M}}$.
The Andrews-Curtis conjecture
Theorem [F. 2017-2021]. The following balanced presentations of the trivial group satisfies the Andrews-Curtis conjecture:
$\bullet ~~\mathcal{P}=\langle x, y~|~ xyx = yxy,~ x^2 = y^{3}\rangle$* [Akbulut & Kirby, 1985]
$\bullet ~~\mathcal{P}= \langle x,y~|~x^{-1}y^3 x = y^{4}, ~x = y^{-1}xyx^{-1}\rangle$ [Miller & Schupp, 1999]
$\bullet ~~\mathcal{P}=\langle x,y~| ~x=[x^{-1},y^{-1}], y=[y^{-1},x^q]\rangle, \forall q \in \mathbb{N}$ [Gordon, 1984]
* First proved by Miasnikov in 2003 using genetic algorithms.
The Persistent fundamental group
Topological inference
Let $\mathbb{X}_n$ be a (possible noisy) finite sample of an unknown topological space $\mathcal X$.
Topological inference
Let $\mathbb{X}_n$ be a (possible noisy) finite sample of an unknown topological space $\mathcal X$.
Goal: Describe (algorithmic) tools to infer topological properties of $\mathcal{X}$ from $\mathbb{X}_n$.

Topological inference
Let $\mathbb{X}_n$ be a (possible noisy) finite sample of an unknown topological space $\mathcal X$.
Goal: Describe (algorithmic) tools to infer topological properties of $\mathcal{X}$ from $\mathbb{X}_n$.


Topological inference
Let $\mathbb{X}_n$ be a (possible noisy) finite sample of an unknown topological space $\mathcal X$.
Goal: Describe (algorithmic) tools to infer topological properties of $\mathcal{X}$ from $\mathbb{X}_n$.


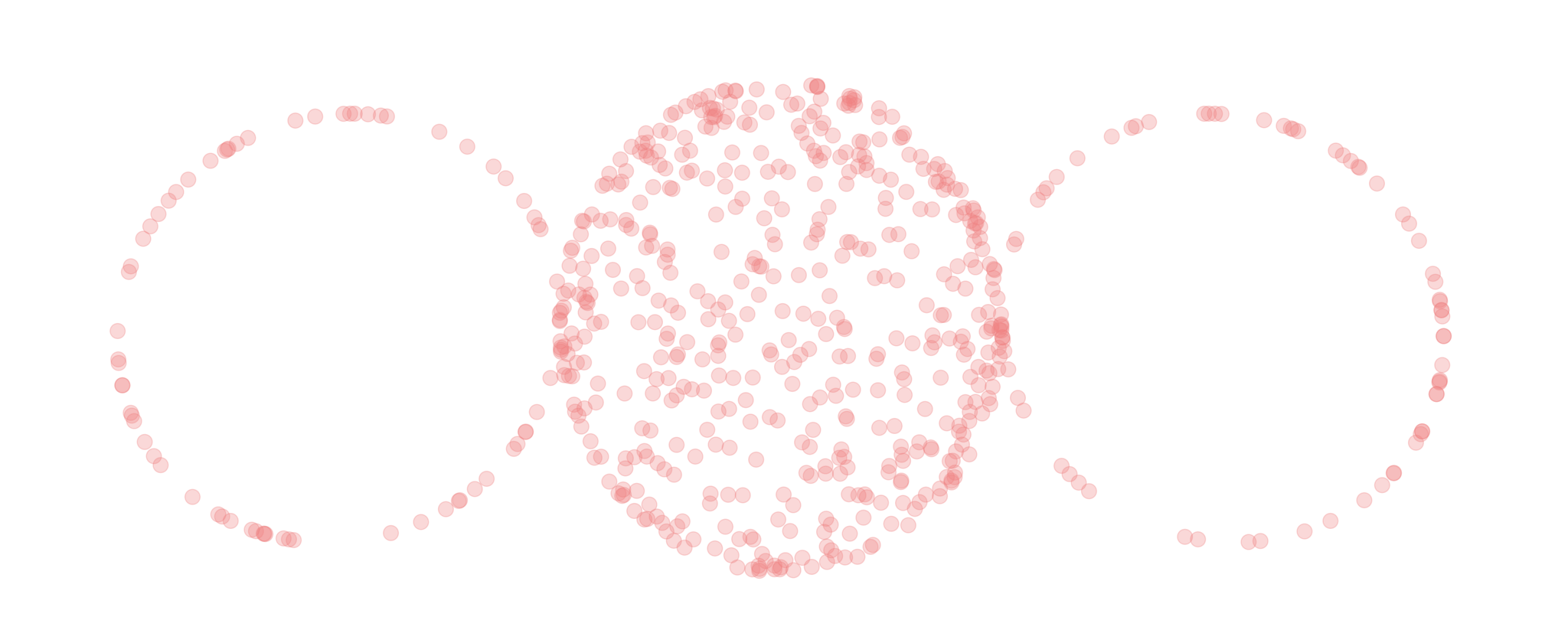
Persistence
- Point cloud $\mathbb{X}_n$
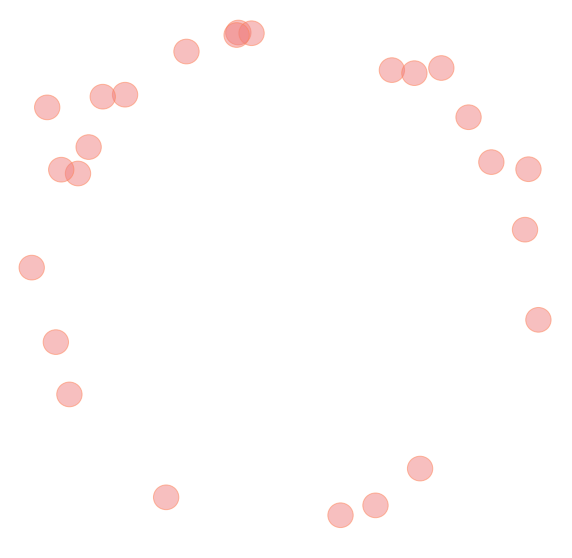
Persistence
- Point cloud $\mathbb{X}_n$

- Filtration of complexes \[K_1 \xrightarrow{i_1} K_2 \xrightarrow{i_2} \dots \xrightarrow{i_{N-1}} K_N\]

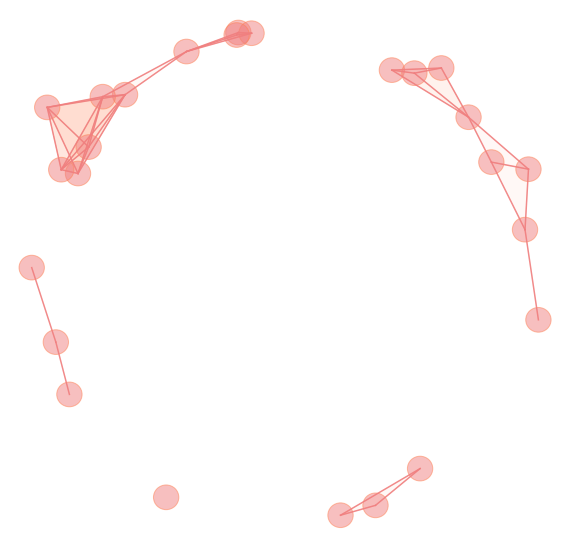
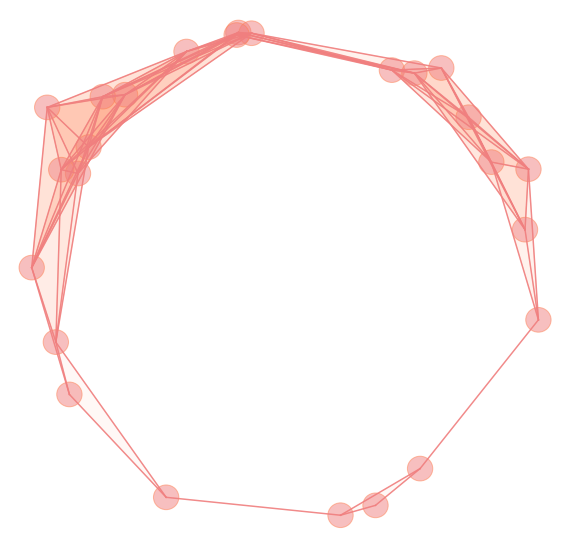
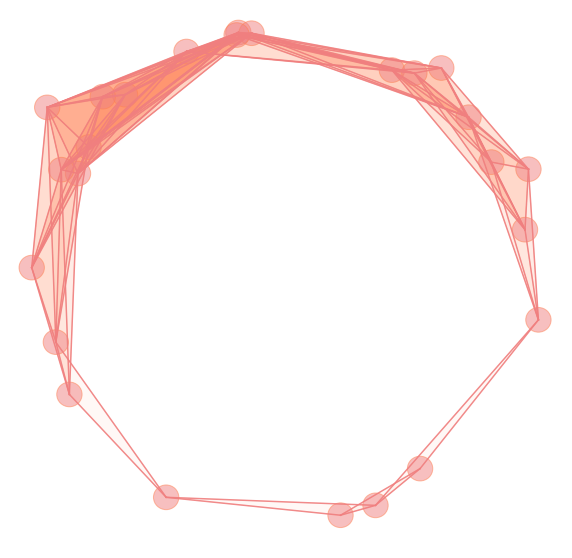
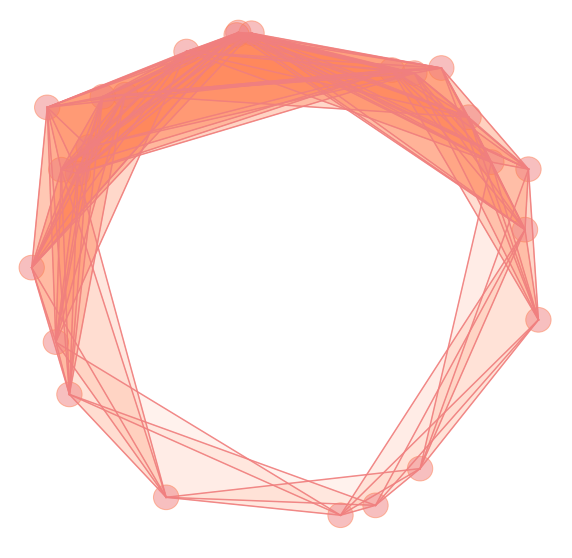
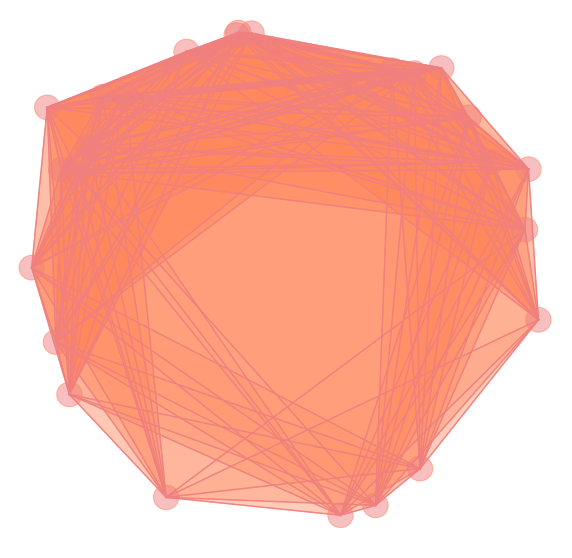
Persistence
- Point cloud $\mathbb{X}_n$
- Filtration of complexes \[K_1 \xrightarrow{i_1} K_2 \xrightarrow{i_2} \dots \xrightarrow{i_{N-1}} K_N\]
- Apply a topological invariant $F$ \[F(K_1) \xrightarrow{(i_1)_*} F(K_2) \xrightarrow{(i_2)_*} \dots \xrightarrow{(i_{N-1})_*} F(K_N)\]






Persistence
- Point cloud $\mathbb{X}_n$
- Filtration of complexes \[K_1 \xrightarrow{i_1} K_2 \xrightarrow{i_2} \dots \xrightarrow{i_{N-1}} K_N\]
- Apply a topological invariant $F$ \[F(K_1) \xrightarrow{(i_1)_*} F(K_2) \xrightarrow{(i_2)_*} \dots \xrightarrow{(i_{N-1})_*} F(K_N)\]
- For every $i < j$, there exist a homomorphism $f_{i,j}:F(K_i) \to F(K_j)$ and the persistent invariant $i\to j$ is defined as \[F^{i\rightarrow j} := \mathrm{Im}(f_{i,j})\]
The persistent fundamental group
- Point cloud $\mathbb{X}_n$
- Filtration of (connected) complexes \[K_1 \xrightarrow{i_1} K_2 \xrightarrow{i_2} \dots \xrightarrow{i_{N-1}} K_N\]
- Apply $\pi_1$ \[\pi_1(K_1) \xrightarrow{(i_1)_*} \pi_1(K_2) \xrightarrow{(i_2)_*} \dots \xrightarrow{(i_{N-1})_*} \pi_1(K_N)\]
- For every $i < j$, there exist a homomorphism $f_{i,j}:\pi_1(K_i) \to \pi_1(K_j)$ and the persistent fundamental group $i\to j$ is defined as \[\pi_1^{i\rightarrow j} := \mathrm{Im}(f_{i,j})\]
- Algorithm?
The persistent fundamental group
Morse reduction. Given $f\colon K_N\to \mathbb{R}$ a Morse function \[(K_1)_\mathcal{M} \xrightarrow{?} (K_2)_\mathcal{M} \xrightarrow{?} \dots \xrightarrow{?} (K_N)_\mathcal{M}\]
\[\pi_1((K_1)_\mathcal{M})\xrightarrow{?} \pi_1((K_2)_\mathcal{M}) \xrightarrow{?} \dots \xrightarrow{?}\pi_1((K_N)_\mathcal{M})\]
The persistent fundamental group
- Point cloud $\mathbb{X}_n$
- Filtration (connected with constant set of vertices) \[K_1 \xrightarrow{i_1} K_2 \xrightarrow{i_2} \dots \xrightarrow{i_{N-1}} K_N\]
- $f\colon K_N\to \mathbb{R}$ a Morse function (with a single critical 0-cell)
- Persistent fundamental group
We developed an algorithm with complexity $O(M^2)$ with $M$ = |2-cells of $K_N$| to compute presentations of $\pi_1((K_j)_\mathcal{M})$ and homomorphisms $g_j: \pi_1((K_j)_\mathcal{M})\to\pi_1((K_{j+1})_\mathcal{M})$ for all $j$ s.t. \[~~~~~~~~~~\pi_1(K_1) \xrightarrow{(i_1)_*} \pi_1(K_2) \xrightarrow{(i_2)_*} \dots \xrightarrow{(i_{N-1})_*} \pi_1(K_N)\] \[~~~~~~~~~~\cong ~~~~~\circlearrowleft~~~~~\cong ~~~~~\circlearrowleft~~~~~~~~\circlearrowleft~~~~~\cong \] \[~~~~~~~~~~\pi_1((K_1)_\mathcal{M})\xrightarrow{g_1} \pi_1((K_2)_\mathcal{M}) \xrightarrow{g_2} \dots \xrightarrow{g_{N-1}}\pi_1((K_N)_\mathcal{M})\]
The Persistent fundamental group
$~O(M^2)$ with $M$ = |2-cells of $K_N$|
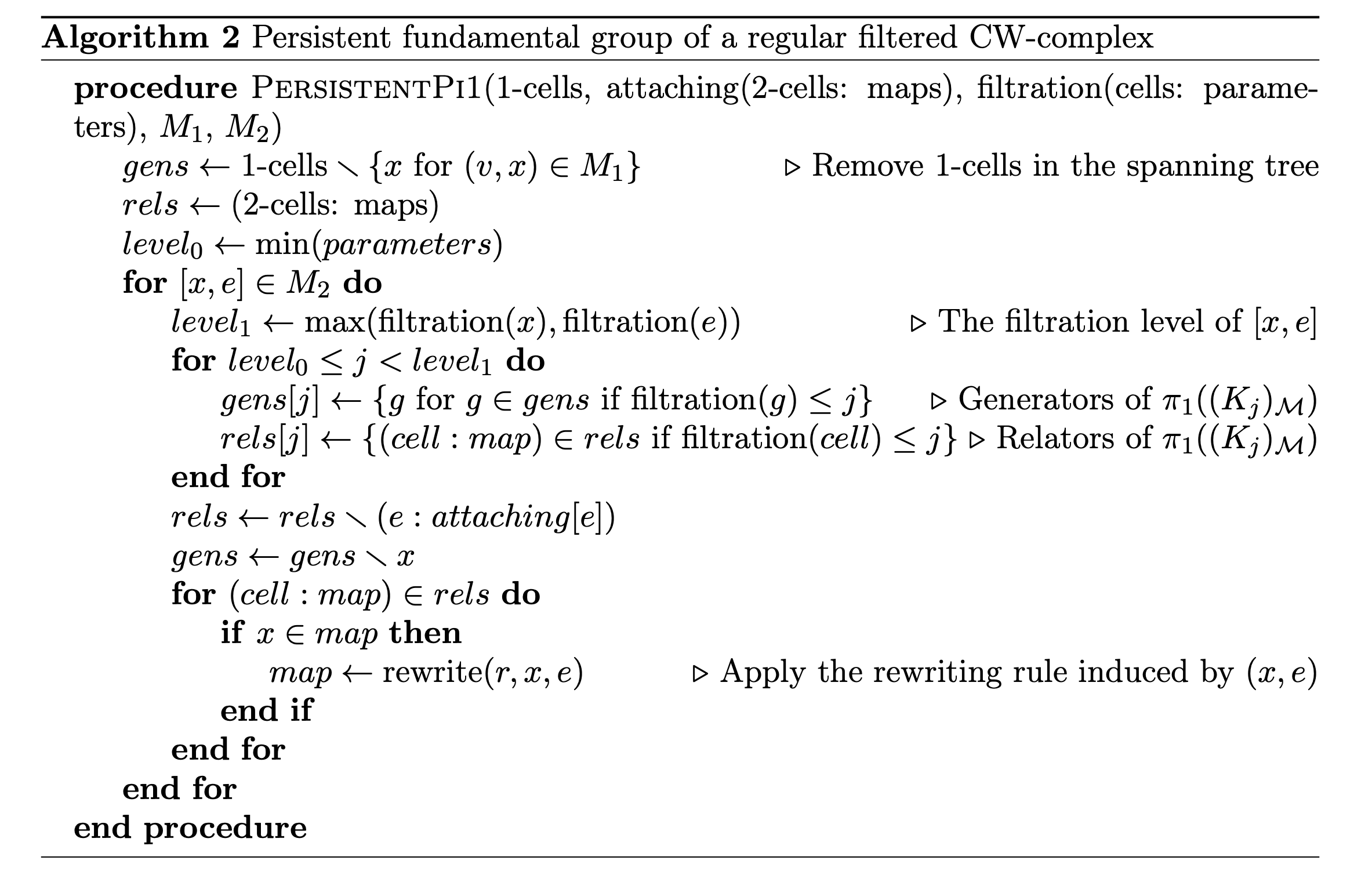
The Persistent fundamental group
$~O(M^2)$ with $M$ = |2-cells of $K_N$|


Example
$S^2\vee S^1\vee S^1$ vs $S^1\times S^1$
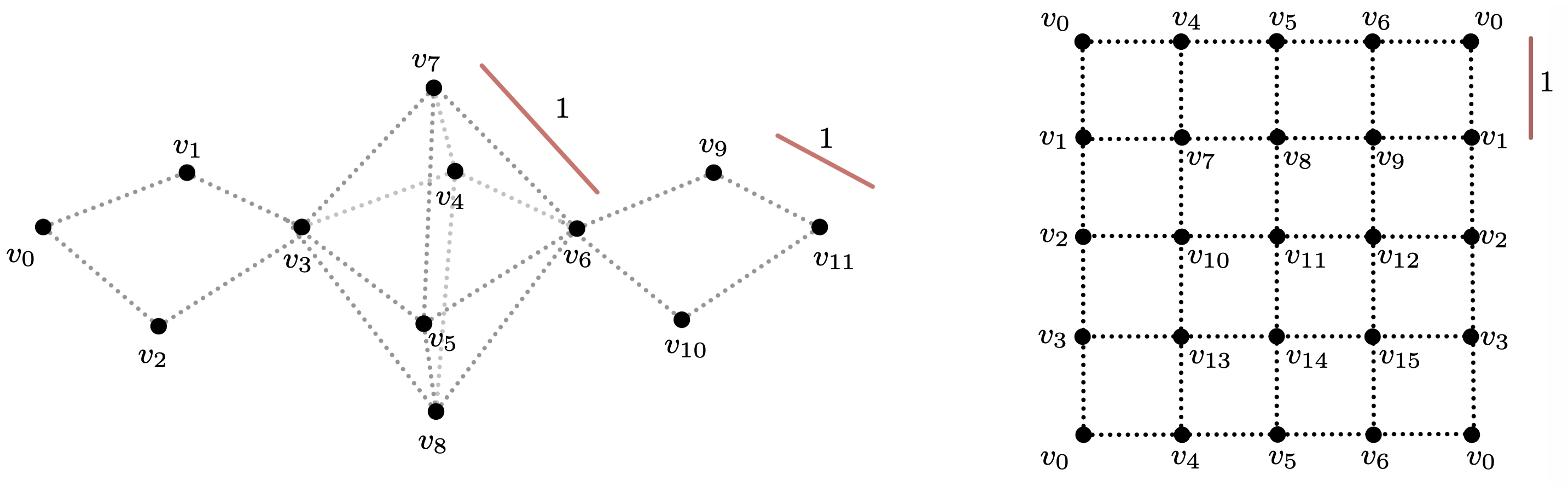
Example
$S^2\vee S^1\vee S^1$ vs $S^1\times S^1$


Example
$S^2\vee S^1\vee S^1$ vs $S^1\times S^1$
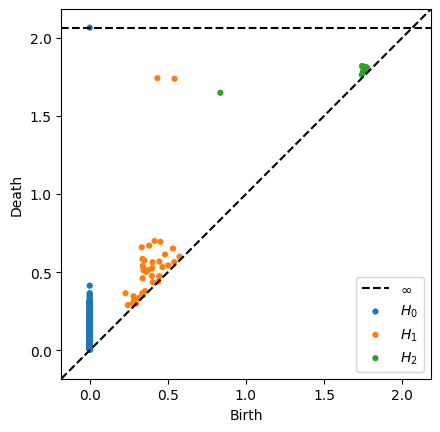
Vietoris–Rips filtration
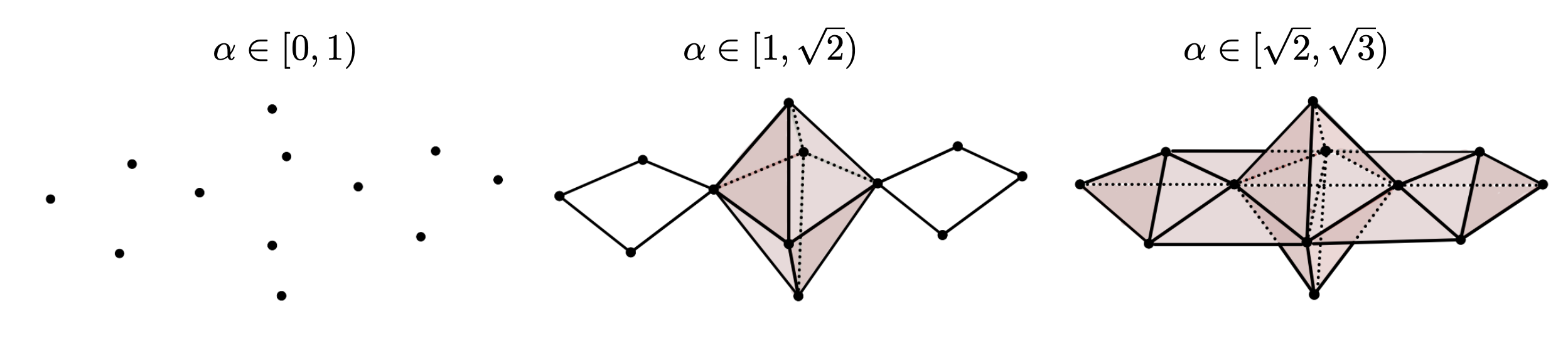
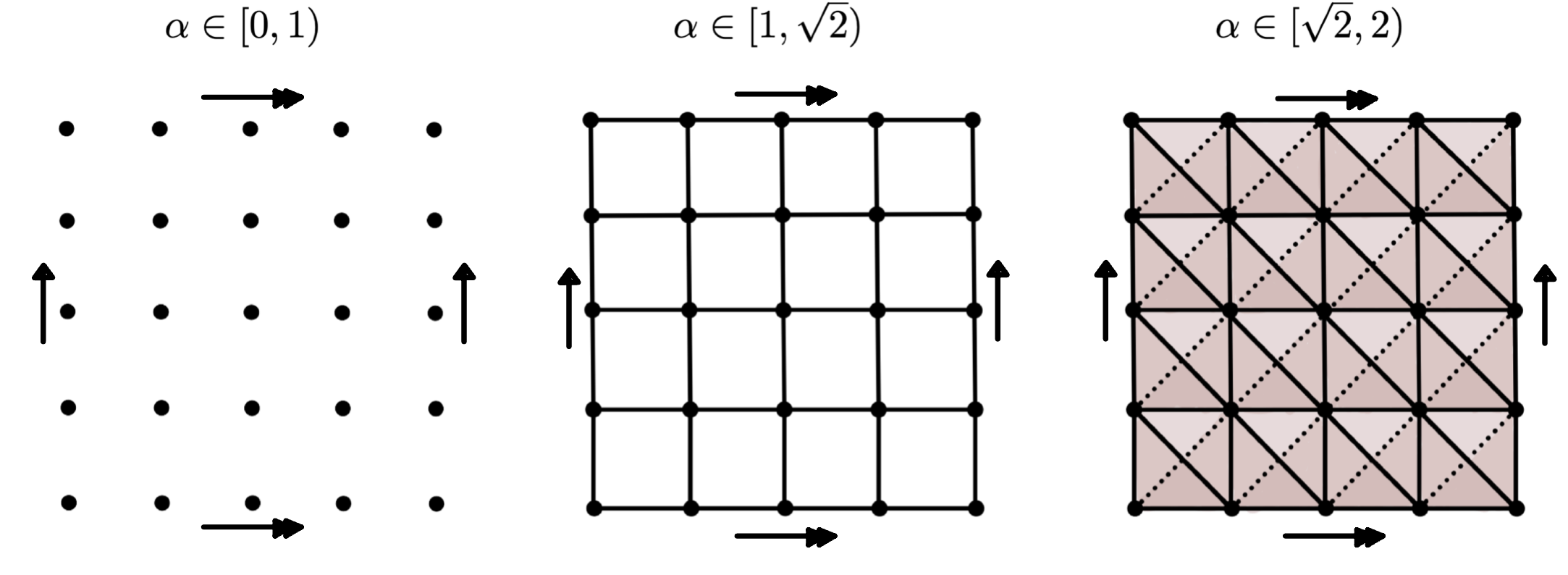
Persistent homology

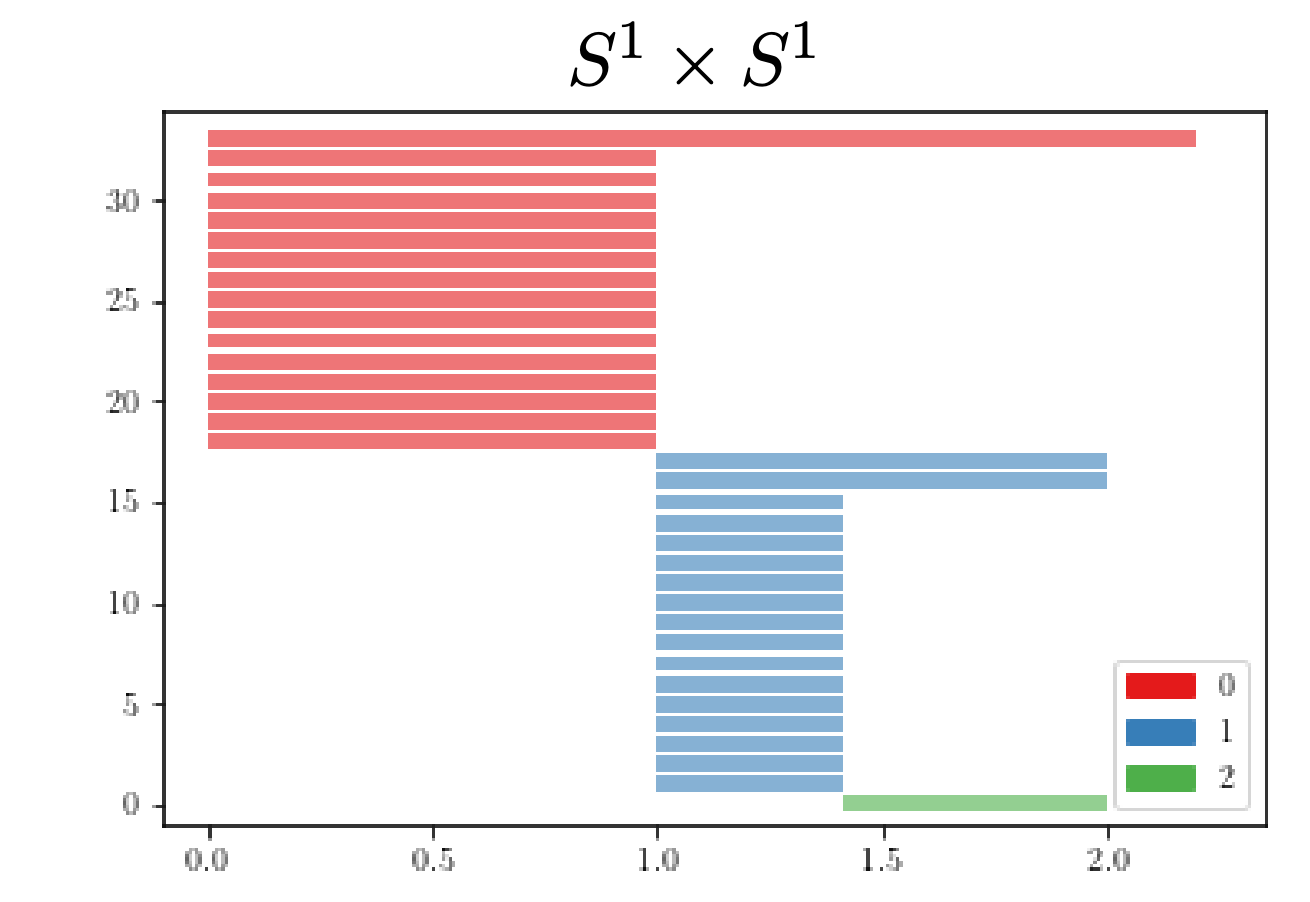
Example
$S^2\vee S^1\vee S^1$ vs $S^1\times S^1$
Persistent fundamental group
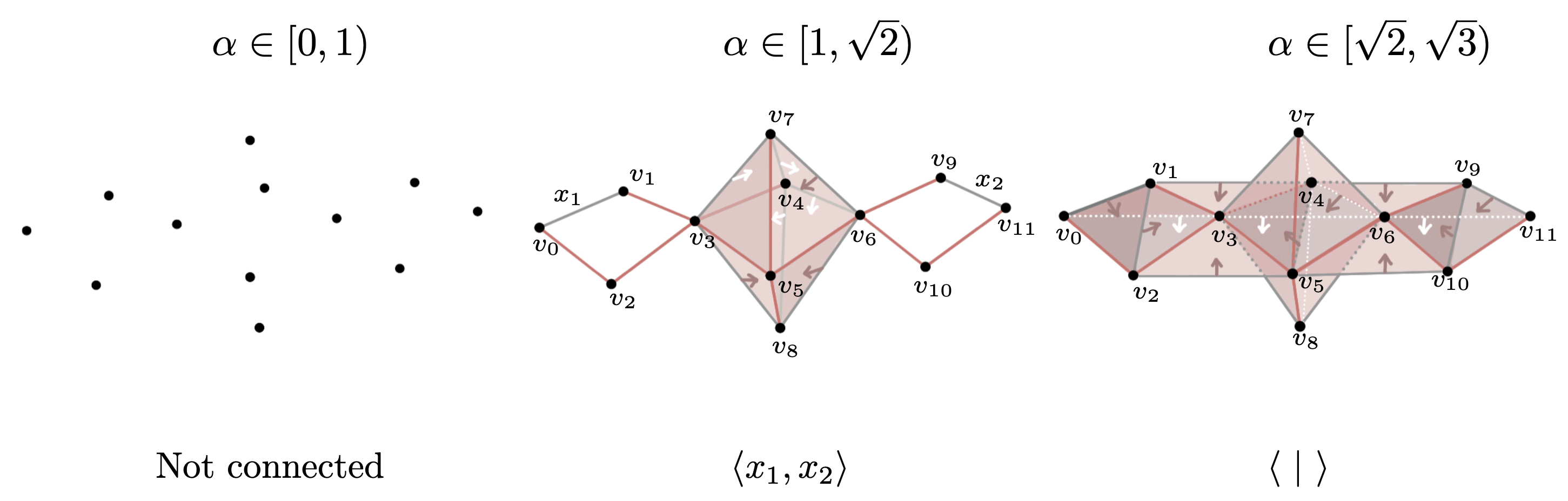
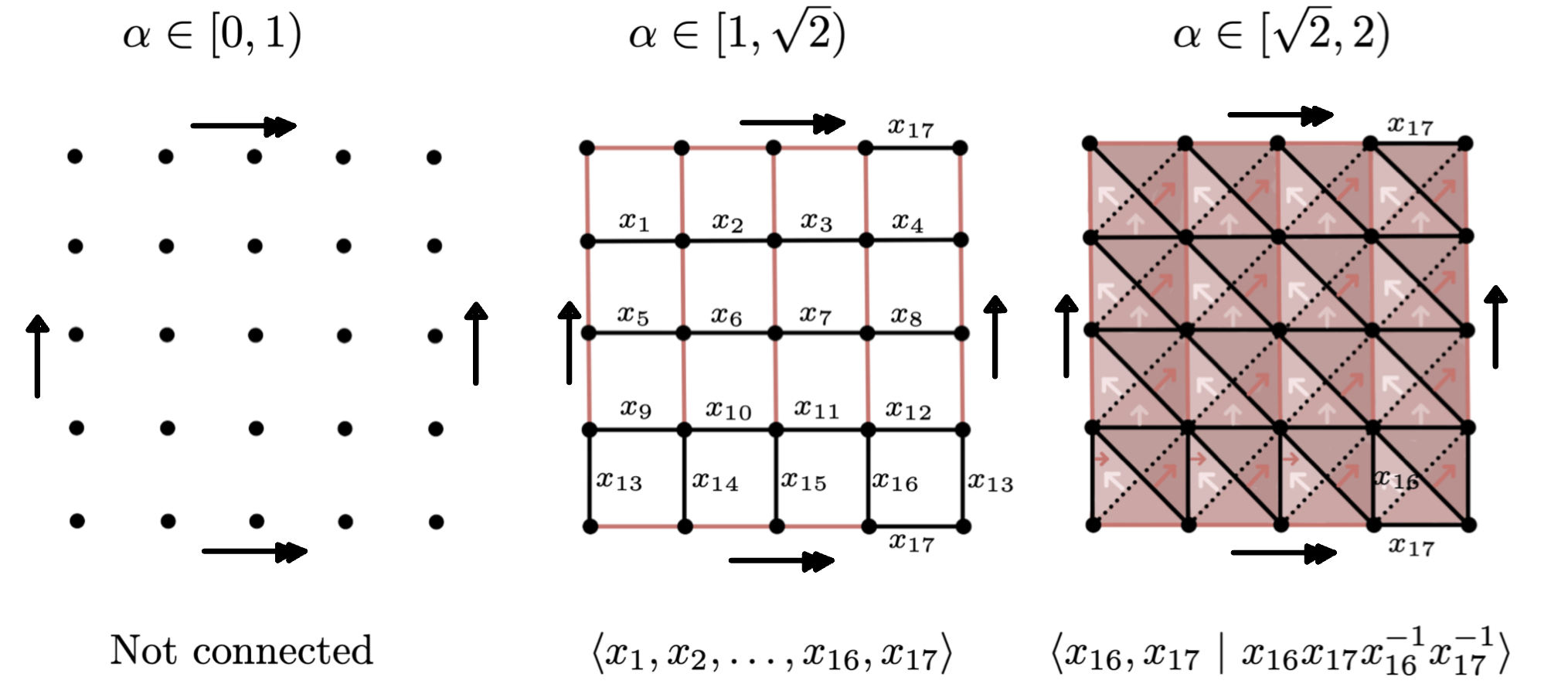
For $\alpha \in [1, \sqrt{2})$ and $\alpha' \in [\sqrt{2}, 2)$, \[\varphi_{\alpha, \alpha'}(x_i) = \begin{cases} x_{16} & \text{if }i = 13,14,15,16\\ x_{17} & \text{if }i = 4,8,12,17\\ 1 & \text{otherwise}.\end{cases} \]
Example
$S^2\vee S^1\vee S^1$ vs $S^1\times S^1$
Persistent fundamental group


Persistent homology


Future work
- Persistence of group invariants.
- Graphical representation (interaction with Persistent Homology).
- Canonical Morse functions for Vietoris-Rips filtrations of point clouds.
- Stability to small perturbations.
- Implementation in GAP & SAGE.
- Applications in real data.
References
- PhD thesis. X. Fernandez, Combinatorial methods and algorithms in low-dimensional topology and the Andrews-Curtis conjecture. University of Buenos Aires, 2017.
- Article. X. Fernandez. Morse theory for group presentations. Transactions of the AMS (to appear) arXiv:1912.00115, 2019.
- Github repository. ximenafernandez/Finite-Topological-Spaces (SAGE)
- Tutorial. Morse Theory 2.0 at AATRN Youtube Channel.
Thanks!
github.com/ximenafernandez $~~~~$ ximenafernandez.github.io/ $~~~~$ ximena.l.fernandez@durham.ac.uk $~~~~$ @pi_ene
Example
$\mathcal{Q}_0 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | ~ x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_1 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12} ~ | ~ x_{12}x_4^{-1}, x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_2 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11} ~ | ~ x_{11}x_4^{-1}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_3 = \langle x_4, x_6, x_7, x_8, x_9, x_{10} ~ | ~ x_{10}x_6^{-1}x_4^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_4 = \langle x_4, x_6, x_7, x_8, x_9 ~ | ~ x_{9}x_4x_6^{-1}x_4^{-1}, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_5 = \langle x_4, x_6, x_7, x_8~ | ~ x_{8}x_4x_6^{-1}x_4^{-1}, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_6 = \langle x_4, x_6, x_7~ | ~ x_7x_6x_4x_6^{-1}x_4^{-1}, x_7^{-1}\rangle $
$\mathcal{Q}_7 = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $
Example

$\mathcal{Q}_0 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | ~ x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_1 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12} ~ | ~ x_{12}x_4^{-1}, x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_2 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11} ~ | ~ x_{11}x_4^{-1}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_3 = \langle x_4, x_6, x_7, x_8, x_9, x_{10} ~ | ~ x_{10}x_6^{-1}x_4^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_4 = \langle x_4, x_6, x_7, x_8, x_9 ~ | ~ x_{9}x_4x_6^{-1}x_4^{-1}, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_5 = \langle x_4, x_6, x_7, x_8~ | ~ x_{8}x_4x_6^{-1}x_4^{-1}, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_6 = \langle x_4, x_6, x_7~ | ~ x_7x_6x_4x_6^{-1}x_4^{-1}, x_7^{-1}\rangle $
$\mathcal{Q}_7 = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $
Example

$~~~~~~~~~P_{(K_1)_\mathcal M} = \langle x_4, x_6, x_{10}, x_{11}, x_{12}~ | ~ \rangle$
$\mathcal{Q}_0 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | ~ x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_1 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12} ~ | ~ x_{12}x_4^{-1}, x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_2 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11} ~ | ~ x_{11}x_4^{-1}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_3 = \langle x_4, x_6, x_7, x_8, x_9, x_{10} ~ | ~ x_{10}x_6^{-1}x_4^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_4 = \langle x_4, x_6, x_7, x_8, x_9 ~ | ~ x_{9}x_4x_6^{-1}x_4^{-1}, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_5 = \langle x_4, x_6, x_7, x_8~ | ~ x_{8}x_4x_6^{-1}x_4^{-1}, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_6 = \langle x_4, x_6, x_7~ | ~ x_7x_6x_4x_6^{-1}x_4^{-1}, x_7^{-1}\rangle $
$\mathcal{Q}_7 = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $
Example

$~~~~~~~~~P_{(K_1)_\mathcal M} = \langle x_4, x_6, x_{10}, x_{11}, x_{12}~ | ~ \rangle$
$\mathcal{Q}_0 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | ~ x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_1 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12} ~ | ~ x_{12}x_4^{-1}, x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_2 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11} ~ | ~ x_{11}x_4^{-1}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_3 = \langle x_4, x_6, x_7, x_8, x_9, x_{10} ~ | ~ x_{10}x_6^{-1}x_4^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_4 = \langle x_4, x_6, x_7, x_8, x_9 ~ | ~ x_{9}x_4x_6^{-1}x_4^{-1}, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_5 = \langle x_4, x_6, x_7, x_8~ | ~ x_{8}x_4x_6^{-1}x_4^{-1}, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_6 = \langle x_4, x_6, x_7~ | ~ x_7x_6x_4x_6^{-1}x_4^{-1}, x_7^{-1}\rangle $
$\mathcal{Q}_7 = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $
Example

$~~~~~~~~~P_{(K_1)_\mathcal M} = \langle x_4, x_6, x_{10}, x_{11}, x_{12}~ | ~ \rangle~~~~~~P_{(K_2)_\mathcal M} =\langle x_4, x_6, x_7~ | ~ x_7x_6 x_4x_6^{-1}x_4^{-1}\rangle$
$\mathcal{Q}_0 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | ~ x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_1 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12} ~ | ~ x_{12}x_4^{-1}, x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_2 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11} ~ | ~ x_{11}x_4^{-1}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_3 = \langle x_4, x_6, x_7, x_8, x_9, x_{10} ~ | ~ x_{10}x_6^{-1}x_4^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_4 = \langle x_4, x_6, x_7, x_8, x_9 ~ | ~ x_{9}x_4x_6^{-1}x_4^{-1}, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_5 = \langle x_4, x_6, x_7, x_8~ | ~ x_{8}x_4x_6^{-1}x_4^{-1}, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_6 = \langle x_4, x_6, x_7~ | ~ x_7x_6x_4x_6^{-1}x_4^{-1}, x_7^{-1}\rangle $
$\mathcal{Q}_7 = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $
Example

$~~~~~~~~~P_{(K_1)_\mathcal M} = \langle x_4, x_6, x_{10}, x_{11}, x_{12}~ | ~ \rangle~~~~~~P_{(K_2)_\mathcal M} =\langle x_4, x_6, x_7~ | ~ x_7x_6 x_4x_6^{-1}x_4^{-1}\rangle ~~~~~~ P_{(K_3)_\mathcal M} = \langle x_4, x_6~ | ~ x_6 x_4x_6^{-1}x_4^{-1}\rangle$
$\mathcal{Q}_0 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12}, x_{13}~ | ~ x_{13}, x_{13}^{-1}x_{12}x_4^{-1},x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_1 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11}, x_{12} ~ | ~ x_{12}x_4^{-1}, x_{12}^{-1}x_{11}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_2 = \langle x_4, x_6, x_7, x_8, x_9, x_{10}, x_{11} ~ | ~ x_{11}x_4^{-1}, x_{11}^{-1}x_{10}x_6^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_3 = \langle x_4, x_6, x_7, x_8, x_9, x_{10} ~ | ~ x_{10}x_6^{-1}x_4^{-1}, x_{10}^{-1}x_9x_4, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_4 = \langle x_4, x_6, x_7, x_8, x_9 ~ | ~ x_{9}x_4x_6^{-1}x_4^{-1}, x_9^{-1}x_8, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_5 = \langle x_4, x_6, x_7, x_8~ | ~ x_{8}x_4x_6^{-1}x_4^{-1}, x_8^{-1}x_7x_6, x_7^{-1}\rangle $
$\mathcal{Q}_6 = \langle x_4, x_6, x_7~ | ~ x_7x_6x_4x_6^{-1}x_4^{-1}, x_7^{-1}\rangle $
$\mathcal{Q}_7 = \langle x_4, x_6~ | ~ x_6x_4x_6^{-1}x_4^{-1}\rangle $